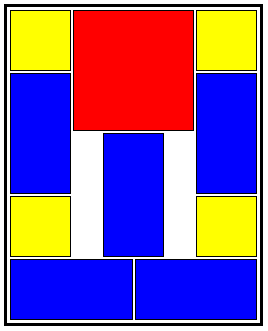
John Conway brought this puzzle to the pure mathematics common room in Cambridge in (I think) the autumn of 1974. It is a sliding block puzzle, a variant on the older Red Donkey puzzle. In a 4×5 frame, we have one 2×2 block, three 1×2 vertical blocks, two 2×1 horizontal blocks, four 1×1 blocks and two empty spaces. The original challenge which Conway presented was to move from

to a position in which the 2×2 block is in the middle of the bottom two rows; counting the initial shift of the 1×2 block a half-space to left or right, this takes just 100 moves. He asked how many moves would be needed to get to the same position reflected top-to-bottom; counting the half-space moves at beginning and end, this takes 151 moves. Counting only positions in which all blocks are regularly set in the 4×5 grid, there are 81100 positions accessible from Conway's starting position. The greatest distance I have found between any two of these positions is 220 steps.
On my own page of diagrams, I offer a variety of alternative puzzles with this set of pieces.
I have also written a program for a 3D printer to make a version of the puzzle which could also be used for the Red Donkey puzzle.
Acknowledgement The diagram is taken from cimt.org.
Back to home page.
31.1.17